Introduction to Astronomy and Motions of the Sky

What's covered here:
What's this all about

What exactly is astronomy? What do astronomers study? They study pretty much everything from the smallest atoms to the entire Universe. That's a pretty wide field of study, to say the least. They can do some rather boring things such as using math and physics to figure out how the Universe and everything in the Universe works - how things formed, how they move, how they live their lives and possibly even how they will ultimately be destroyed. They look at how things move, and unlike meteorologists, they are able to predict things, such as when the Sun will rise, when the Moon will set or a whole slew of other things. Some astronomers spend all of their time gathering images of objects in the sky using a wide range of instruments. Of course, there is not a lot that they can do on cloudy days - though you'll see there are exceptions to this rule. Astronomers study such things as planets, stars, galaxies, moons, asteroids, comets, space dust, gas between the stars, groups of galaxies, black holes, the shape of the Universe and ultimately the fate of the Universe.

If you want to really get an astronomer mad at you, just mention astrology. This will not win you many friends in the astronomical community. However, like an old skeleton in the closet, we (astronomers) can't ignore some of the concepts that are usually associated with astrology. Of course, if you want to make a lot of money from a bunch of gullible people, become an astrologer - I wouldn't recommend it, but it can't be denied that astrologers can make some big bucks, mainly because people are just too easily boondoggled. Just remember, if you ever use the word astrology in class when you actually should say astronomy, I just might have to make you stand in the corner.

The history of astronomy is pretty long; in fact, you can think of astronomy as the oldest of all of the sciences - not to be confused with the oldest profession! In spite of this long and noble lineage, we can't ignore the linkage between astronomy and astrology. In fact, in the old days, astrology was even considered a serious (!) topic of study, along with astronomy. Both were important, at that time, in trying to figure out how the sky operates. Why was it important that we knew how the sky operated?

In the old days, and I don't mean 1974 by "old," before the invention of such annoying things as digital watches, cell phones and other modern bits of technology, people's lives depended upon the sky. Remember, most people in the old days were not formally educated, so they might not have been able to understand a clock or a calendar (reading was generally only for the rich and wealthy, and those who worked for them). Most people lived in an agriculturally based society - not too different from Iowa, but even more rustic. What does that have to with astronomy? In those days, it was important to know when to do things - remember, most of the people wouldn't know a calendar from a hole in the ground - well, maybe they would if they fell in the hole, but you know what I mean. Any ways, it was important for the people to know when to do the things that were vital for their existence. Since most people were farmers, they needed to know when to do such important things as plant the crops, harvest the crops, expect the winter to come to an end, and check to see if you still had enough food left to survive. These were pretty basic things, and there was no CNN to tell people what to do and when to do it.

What does planting, harvesting and such have to do with astronomy? By knowing that a certain constellation was visible in the evening sky (or the morning sky), people knew it was time to harvest; they knew that at this time of year, the rivers would tend to flood; or by noting the position of the Sun at noon in the sky, they knew that it was a month until the end of the cold weather - killing frosts. Of course, people, being rather uneducated, were also under the belief that the stars and planets in the sky would also effect their lives just by their locations in the sky. Yes, this is what that other "a" word (astrology) is all about. Since people were so dependent upon the motions of objects and the predictable patterns in the sky (astronomy) to stay alive, if they could use another aspect of the sky (astrology) to hedge their bets, then it perhaps helped them to survive. Generally, astrology never helped anyone, except for the astrologers who got rich off of all of the fools that believed them.

Also, other aspects of the sky were important, and are still important today. Do you know what the phase of the Moon is today? Do you think that is important? You probably don't think it is, but several major cultures still use the Moon as a basis for their calendars - these include people who use the Islamic,  Judaic, or Chinese calendar. That's a pretty large number of people. You still think that you don't care about what the phase of the Moon is? You don't think it's important for the typical Iowan? Here's a question - how is the date of Easter determined? You know it is on a Sunday, but the date changes from year to year. Why? I'll tell you - it depends upon both the Sun and the Moon. The day that Easter is celebrated on is the first Sunday after the first Full Moon after the first day of Spring (also called the Vernal Equinox). You can go look it up - you'll see that this is how it is set up! The first day of Spring is based upon the location of the Sun in the sky, so that and the phase of the Moon determine the date of Easter.

In the old days, it was important for people to know how the objects in the sky moved and when they were in certain locations. What are you going to do if it is cloudy all of the time? How would you know when to plant your crops if you can't see the stars, the Sun, the Moon, or whatever object you use as a calendar? What then? This is sort of where the science (and math) comes in. Astronomers not only can tell where things are, but are also able to predict where they should be. In this way, they can tell people when to do their agricultural work at the right time, regardless of the weather. Here's something you might not have known - in western Europe, most of the people who did astronomy (and astrology) were employed by the Christian church, since they needed to know when various festivals or celebrations had to be held. People working for the church, such as the priest and monks, tended to be literate and were able to do complex calculations that showed how the objects in the sky moved. If you wanted to know when you were supposed to do various things, you needed to know when things were going to be at certain locations and to do that you had to figure out how everything worked - and this isn't easy.

Of course, if you were to make your fortune suckering people with astrology, then you also needed to know math and the workings of the sky, since you had to know where things were or will be in the future. If you can figure out the motions of the planets along with the Moon and the Sun, then you can really make some serious money in the astrology trade, so even the non-scientific astrologers did have to know enough science to cast their horoscopes. Of course, nowadays, astrologers can be total idiots since a computer can figure out everything for them.

Now that I've convinced you about the importance of astronomy in the old days and how it used to be linked with astrology, let's just see how really tricky it is. What were those complicated motions that your friendly neighborhood astronomer had to predict and understand?

Motions of the Sky

First a word of explanation - when we talk about stuff "rising" and "setting" it is the appearance of something rising or setting. In reality objects like the Sun or Moon aren't physically rising from the horizon. Most of the motions we see in the sky are caused by our (the Earth's) motion, both its rotation and orbital motion. It is just common to say "the Sun rose in the East today" rather than to say "the Earth rotated toward the direction of the Sun, which caused it to appear to rise in the east". So all of the "motions" described below are really apparent motions - not really an indication of how things are actually moving, but how they appear to move.

Motions/Events of the Sun

The Sun is pretty bright, and without it, all life on Earth would be nonexistent, so let's see how complicated its motions are. We'll just consider the motions that you would see in Iowa - If I were to ask you to come up with a method that would allow you to determine the exact rise or set time of the Sun for, say, May 17th, could you do it? This is actually a pretty difficult problem and we won't tackle it (yes, you can breath a sigh of relief now), but there are some other things you'll have to predict - just stay tuned.

Motions/Events of the Moon

After the Sun, the Moon is the most obvious thing in the sky. Even on hazy or semi-cloudy nights, it is often possible to spot the Moon, so what are its motions that people have to worry about? In a way the motions of the Moon are even more complicated than the Sun's motions. There are some aspects of the motions that are pretty easy to understand, as you'll see.

Motion of the Stars

The other main players in the evening sky are the stars, and I don't mean Brad Pitt or Angelina Jolie, but those twinkly little things in the sky. What do we know about them just by looking at them with our eyes? Of course, if you have looked carefully at the stars, you have noticed that they have different appearances - some stars are brighter and some are fainter. If you have good eyes and look at stars carefully, you may also notice that the colors of stars vary - this is particularly easy to notice with the really bright stars. Some stars are bluish-white, others are yellow, and others are red.

Motions of the Planets

With your unaided eyes (that means without using binoculars or a telescope) you can see six planets (can you name them all?). How can you tell that an object in the sky is a planet and not a star? I'll tell you after I go over the motions of the planets (yes, I do like to keep my students in suspense!). Have you figured out the six planets that you can see with your naked eye? Did you remember to count the one that you live on?

Models of the Sky - Celestial Sphere

All of the motions that I went through are pretty complex, and they all need to be explained, so that you can predict the motions, so that you can set up some sort of calendar system, so that you'll know when to celebrate your various festivals and celebrations or when to plant or harvest your crops, or, well, whatever you need to know. To explain all of these motions, early astronomers/astrologers needed to figure out the mechanics of the sky - they needed a model (something that could explain the motion; not necessarily a physical model, but at least something that they could write down). Since the motions of the stars are fairly uniform, the model for their motions is easiest to do and we'll tackle it first. One of the concepts that people believed in the old days was that the stars (and everything else in the sky) were moving and the Earth wasn't. This sort of made sense, mainly because people did not feel any motions from the Earth - they had no sense that it was moving, like you would feel motion on a ship, a horse or a wagon. Obviously to these people, the Earth didn't move. Now we know that this idea is totally bogus, but in the old days it made sense, and it also helped astronomers to figure out how to make a working model of the sky.

The early, simple model of the sky is known as the Celestial Sphere. What is it? Basically a big see-through imaginary globe around the Earth. The stars are stuck on this sphere, and as the sphere spins around, the stars would move with it. This really explains how the stars move pretty clearly, since they are on this spinning globe, those near the axis of the rotation, like near the North Star, would make little circles around the pole star, while those further from the pole would move along larger paths across the sky. If you don't get this idea, think about how an umbrella looks as you spin it around above you. If you have a bunch of spots on the inside of the umbrella, the spots far from the handle will make large circles around as you rotate the handle, while those near the handle will make little circles. If you were to take the ends of the umbrella and stretch them around you would have a sphere, just like a Celestial Sphere surrounding the Earth. People thought that the stars' patterns never changed (they stayed in the same constellation patterns) since they were all "stuck" on this big sphere. Stars also never appeared to change their appearances, their colors or their brightnesses - at least not in a way that was noticeable. By putting them on a fixed, big sphere, people sort of made them eternal. The worst part of it all is that this model worked really well!  BUT IT IS TOTALLY COMPLETELY WRONG!!! One of the obvious problems is that it implies that all of the stars are at the same distance from the Earth, and this is not correct. Even though this model of the sky is not correct in terms of the real Universe, it is useful in determining positions of objects and defining a coordinate system of the sky. Just remember - IT IS WAY WRONG!!!!

The constellations that the stars are associated with used to just be traditional groupings. Different cultures had different constellations. When astronomy got more scientific and organized, the little stick figures you see for the constellations sort of changed over time. Today there are 88 constellations in the sky and they are actually sort of like counties in a state. There are 99 counties in the state of Iowa and each piece of land in the state is part of some county, based upon where the boundaries are drawn. This is also how we now divide up the sky - the constellations don't just define a stick figure of a dog, horse or person, but a region of the sky. Something in that region belongs to a certain constellation. This is shown in Figure 1.

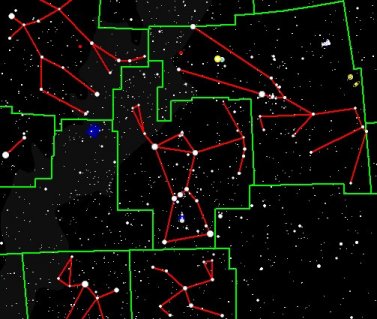Figure 1. The territory that belongs to a certain constellation is shown by the green lines, while the red lines show a traditional way of drawing the "shape" of the constellation. The constellation boundaries are not subject to change, but the lines that represent the stick figure for the constellation can be drawn in any sort of pattern.

Even though the Celestial Sphere is not a real model of the sky, it is useful for mapping out locations of objects in the sky.  The Celestial Sphere was set up so that it used some of the coordinates that are used on the Earth. Remember, the Earth was thought to be at the center of the Celestial Sphere, so it wasn't too difficult to extend the Earth's coordinates to the sky. Putting the Earth in the middle of everything may seem egotistical, but there were other reasons for doing it. Figure 2 shows the basic set up for a Celestial Sphere. There are a couple of special locations that need to be pointed out.

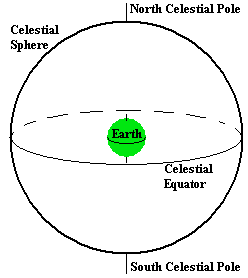Figure 2. The Celestial Sphere, an imaginary ball around the Earth upon which the stars were thought to be located. This is not true - the stars aren't on this sphere, but it does provide an easy way to map out the sky.

Celestial Poles - these are points on the Celestial Sphere that are directly above the Earth's Poles, so there is a Celestial North Pole and a Celestial South Pole. You can also say North Celestial Pole and South Celestial Pole; it doesn't really matter how you say it.

Celestial Equator - this is just a line that is directly above the Earth's Equator. Like the Earth's equator, the Celestial Equator goes all the way around the Celestial Sphere.

We need to simplify some locations in the sky now. We can talk about stuff that is directly over head, or we can say stuff is at your zenith - this just means the location right over your head. You have a personal zenith and what is there depends upon where you are on the Earth, what time of day or night it is and what time of the year it is. There is also a term for the exact opposite of zenith, but we don't really care about that since it would be in the direction of the ground and there are no stars visible down there.

Another special direction is the horizon, though that is not really one particular direction, but sort of, well, your horizon. To be kind of technical, the ground meets the sky at the horizon, and generally, there are 90º between the horizon and the zenith (especially in Iowa). If you do want to get specific about the horizon, you could say the southern horizon, the northern horizon, etc.

Here's a rather surprising concept that you might not have known about - the ancient astronomers did not, I repeat, NOT, believe that the Earth was flat. In fact, it was pretty much agreed that it was spherical, though the size of the sphere was so big that to us puny humans it looked like a flat surface. Actually, as you'll see, ancient astronomers were sort of fixated on everything being spheres. We'll get to that later.

As previously mentioned, the Celestial Sphere was useful for finding your way about the sky, since like on a globe of the Earth you can designate various coordinates to measure locations or positions of objects. First, we'll tackle the north-south coordinate system. On the Earth, the amount that you are north or south of the Earth's equator is measured in degrees of latitude. There are certain special locations on the Earth. If you are at the Equator, you are at a latitude of 0º; if you are at the North Pole, you are at a latitude of +90º or 90º North (this is the highest possible value) while at the South Pole your latitude would be -90º or 90º S.

We're not at one of those locations - we're in Cedar Falls (at least I am). How do we determine our latitude? Simply draw a line from our location to the center of the Earth and a line from the Equator to the center of the Earth (see Figure 3). Now measure the angle between these two lines - this is our latitude! For Cedar Falls, our latitude is 42.5º N. The latitude of any location on the Earth can be found by measuring its angular distance from the Earth's equator. Draw a line from the North pole to the center of the Earth and you'll end up with an angle of 90º - which is the latitude of the North pole!

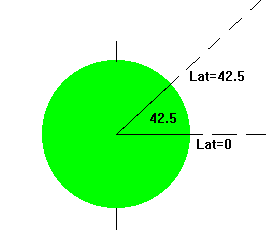Figure 3. The definition of latitude. The angle between your location and the equator (where latitude =0) determines the value of your latitude.

Now, if we were to take this coordinate system and stretch it up to the sky, we would be cooking! Why can we do this? First of all, the Celestial Sphere is already sort of set up the same way as the latitude system - there is a Celestial Equator and there are Celestial poles. Just extend the latitude system to the Celestial Sphere to get the angles that objects are North or South of, not the Earth's equator, but from the Celestial Equator. We can't call this system latitude, since that name is already taken. Instead, this coordinate system is known as declination (abbreviated as dec). Declination is just the angle an object on the Celestial Sphere has as measured from the Celestial Equator, just like latitude is the measure of the angle between an object on the Earth's surface and the Earth's equator.

One really neat thing about this system is how declination and latitude are linked. An object at your zenith (remember, that is right over your head) will have a declination value equal to your latitude! If you are located on the Earth's equator (0º latitude), at your zenith would be a declination of 0º (which is the declination of the Celestial Equator). If you are at the North pole, you are really cold, and your latitude is 90º N. If you don't freeze to death, you might notice that at your zenith is a declination of 90º N. If you are in Cedar Falls, 42.5º N latitude, then at your zenith is a declination of, you guessed it, 42.5º N. Check out Figure 4 to see this sort of arrangement.

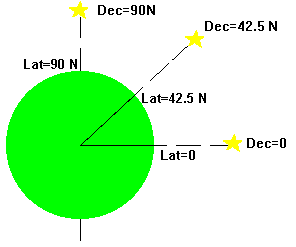Figure 4. How declination and latitude are related. An object at your zenith has a declination value that equals the value of your latitude.

Just like latitude, declination is measured in units of degrees. The two extremes are at the North and South Celestial poles: +90º to -90º respectively (or you could say 90º N and 90º S). You can't have a declination greater than +90º or less than -90º!

Sometimes a degree is pretty big and you need to measure an angle that is much smaller than a degree, so you need to use a smaller unit of measure (sort of like the way an inch is a smaller unit of a foot). To make life easier, we can divide one degree into smaller units known as minutes. To be precise, 1º = 60' (the dash stands for minutes). Sometimes using minutes is not enough; even smaller units are needed. We can divide each minute up into (you guessed it) seconds. Of course, the division is 1' = 60 " (two dashes signify seconds). For those with nothing better to do, you might note that 1º = 3600". An object's declination can be given very precisely as, for example, -34º 27' 41''.

A lot of times astronomers have to keep track of time (not that we ever have any dates or something like that, but just to keep track of events in the sky). If astronomers need to talk about minutes and seconds, like as in units of time, how can we know that they are talking about time units and not angle units? To distinguish between angular minutes and seconds and time minutes and seconds, the word arc second or arc minute is often used. You could say that there are 3600 seconds in a degree, or you could say that there are 3600 arc seconds in a degree. By using arc seconds people would know that you are talking about angles and not time.

I should mention that angles are used for not just positions but also relative positions and angular sizes. What's that mean? You could say that one object is 10° from another, or you could say that an object is 10° wide. These are two different things. One is how far apart two objects appear in the sky (their actual separation may be quite different). The other is how big an object appears. This is basically the amount of sky that an object covers. Two big astronomical objects that you can see with your eye are the Sun and the Moon. How many degrees across do you think they are? 5°? 10°? Well, you might be surprised to learn that both are about 1/2° in size! That's pretty tiny! You don't believe me? Well, take your thumb (you have a thumb don't you?), hold it at arm's length and place it over the Moon (this is less painful than holding it over the Sun). Can it cover the Moon? Your thumb is about 2° across when held at arm's length. Also try it using a pencil held at arm's length. You might be surprised.

We're going to divert for a moment from the discussion of the celestial sphere and all that to talk about angles, sizes and distances. I mentioned that your thumb held at arm's length is about 2° wide. Why did I have to say "held at arm's length"? What happens if you move your thumb really close to your eyes? If you are pretty normal, you'll notice that your thumb looks really big. Does that mean it has a larger angular size? Yes; your thumb's angular size is now larger. What does that actually mean? It means that it covers a larger region of your field of view - it appears bigger. If you could move your thumb further away, it would look smaller - it would have a smaller angular size (basically be less than 2° in size). Is your thumb actually changing its size? No, of course not - not unless you hit it with a hammer during this process.

This is all rather cute and silly, but what does this have to do with science and all? Actually, there is a relation between the size of an object, the distance the object is from you and its angular size. The relation between these things is

S = R 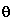 0.0175

where S is the actual size of the object (how wide it is), R is the distance of the object from your eyes and 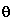is the angular size (how many degrees wide it is). This nifty little formula is known as the Small Angle Formula, since it really only works well for small angles (less than 10º). One of the neat things about this formula is how these quantities are measured. R and S are in the same units - by this I mean that if R is measured in inches, then so is S; if S is measured in kilometers, then so is R; if S is measured in pickles, then so is R; and so on.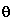 is measured in degrees - you should know what those are; at least, I hope you do. The number in the formula is there for making sure everything comes out properly in the end (so that you end up with the correct units).

If you want to actually use this formula, you need to know two out of three of the things in the formula. For example, if you know that the Moon is 1/2º in size (= 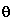), and it is 3476 km wide (=S) can you then determine how far away is it? Just take the formula and determine the value of R (the distance). The formula is

R = S /(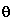 0.0175) = 3476 km / (0.5 x 0.0175) = 397,257 km

The Moon is 397,257 km away.  Basically, if you know two of the things, you can always get the third. This is one way to determine the actual size of an object so long as you know the angular size and the distance. Conversely, you can get the distance if you know the actual size and the angular size, or you can get the angular size if you know the distance and the actual size.

We'll now go back to the coordinates discussion.

How about the East-West designations? We used latitude for the North-South system, so let's see if we can do the same with longitude for the East-West coordinates. Unfortunately, we can't use the longitude system since the objects in the sky move too quickly - they aren't located constantly over the same location on the Earth. Instead, astronomers use a system based on a clock. This is logical since it appears that the sky gets back in the same configuration after 24 hours (again, you should remember that this is only how it appears; the sky isn't really rotating!). Astronomers divide the sky into 24 units known as hours, which go all the way around the Celestial Sphere.

To make your life complicated the name for the East-West coordinate system is known as Right Ascension (R.A. for short). Values of R.A. increase as you go further to the east. There is a location labeled 0h; further east is 1h, then 2h, ... and 22h, then 23h, finally 24h which is actually the same as 0h. This set up is similar to how military time is given - numbers increase as you go east until you are back to where you started from. If you need more precise units, 1h of R.A. can be divided up into 60 minutes (1h= 60m), and one minute of R.A. can be divided into 60 seconds (1m=60s).

Note the units of R.A. and dec are different, R.A. has h m s, while dec has º ' " . An object's full coordinates could be something like 2º 33' 17'', 14h 7m 33s - not that you need things so precise all of the time, but if you need to be that precise this will help you not get lost. Every object in the sky, not just the stars, but also the planets, the Moon, the Sun and everything else - can be located at a distinct value of declination and Right Ascension, just like every location on the Earth can be designated according to a set of latitude and longitude coordinates. Here is a handy little java program that allows you to click on a map of the sky to see the coordinates - I've only included the degrees and minutes values for declination and the hours and minutes for RA since it is sort of a rough map. One of the things you'll see is how the RA increases in value as you go toward the left, which is East in this view.

Star Maps

Often people want to be able to find objects in the night sky, either because it is a new event, like a comet or supernova, or they just want to know where certain stars or constellations are located. You can certainly get an atlas of the night sky which is based upon the the celestial sphere model. Such maps would have the grids of RA and dec clearly indicated, but such maps may be too much - too detailed. What if you just want to look at the Big Dipper or find Jupiter? There are various on-line planetarium programs, as well as apps you can download that can show you what is in the sky, but the classic type of star map is that which can be printed out and used in the field. These can be found at a variety of sources including Skymaps.com. These maps take the entire sky and compress it down to a sheet of paper. Obviously there will be a bit of distortion in the image. Also since it is a map of what you see when you look up, rather than a map of the Earth in which you are looking down, the cardinal directions on the map are rather confusing.

Sky maps that show the entire sky usually indicate the time of day and days of the year during which they are closest to the sky in appearance. Objects at your zenith are at the center of the map, while along the edges are the various horizons - north, south, east and west. Often the Milky Way's path is shown, as well as the locations of planets and non-stellar objects that could be observed with a telescope. If you look at several different star maps, you'll see that the way that the constellation stick-figures are shown varies slightly - again, the stick figures don't define the constellation, so there are no rules concerning how they should be drawn.

Objects on the star maps, such as stars and planets, are also shown using dots of different sizes. The larger dots indicate that the object is brighter, which makes sense. Something that doesn't make sense is the manner in which astronomers quantify a star's apparent brightness. Often you'll see a scale showing the dot size and a value labelled as magnitude. You'll notice that the smaller magnitudes, even the negative values, correspond to the larger dots. That's something we'll get to in more detail later - a small value of magnitude indicate a very bright object, while a large value indicates a very faint object. Yes, that is screwy, but that's the way that astronomers have set things up. Go figure.

Positions of objects relative to the horizon

If you were to sit outside on a clear night, you would notice that over the course of the evening different objects pass over your head, and other objects will appear to rise and others will appear to set. If you were to go outside a month later you would see stars still doing these things, but the stars wouldn't be in the same places doing the same things at the same times as you saw them a month ago. A star that was located near the eastern horizon last month might now be very high above the southeast horizon at the same time of night. A star you saw in the west might no longer be visible. When and where stars are located in the sky varies from night to night. Remember, different constellations are visible at different times of the year - you'll see why this is in the next set of notes.

Perhaps you want to see an object when it is highest above the horizon, as far from the ground as possible. This is the best time to see an object, since there is less chance that it will be obscured by trees or buildings, and the atmospheric haze is generally less as you get further above the horizon. When is an object located furthest from the horizon? We don't mean just when an object is at your zenith, since not all objects will pass directly over your head.

The best time to view an object is when it is on your meridian. Like the zenith, the meridian is a special location, though it is not just a single point. It is actually a line that runs north-south and passes through your zenith. When objects are on your meridian, they are at their "highest" location in the sky. You are most familiar with this when you see the Sun high up in the sky. It is highest above the southern horizon at about noon. At this time, it is directly due south of you. If you were to watch any star or object in the sky and measure its height above the horizon, you'd notice the largest angle it has above the horizon is when it is on this north-south line. Check out Figure 5 for an illustration of this. Another feature of the meridian is that all objects on your meridian have the same value of R.A. (this is because it is a line that runs exactly North and South  - no part of it is further to the East than any other part of it). This is the same way that a line of longitude (which, like the meridian, runs north  to south) acts - all objects on that line have the same value of longitude.

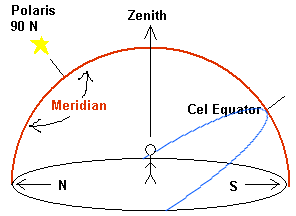Figure 5. Meridian line goes from north to south and passes through your zenith. Objects on the meridian are at their highest elevation above the ground. If there was a star on the Celestial Equator, you would see it rise in the East, be highest above the southern horizon (when it is on your meridian) then get lower to the ground before it sets in the West.

Now we're going to do some fun things with objects on your meridian - and believe me, you'll want to pay close attention to this stuff since you can be guaranteed that it will be on the test. Let's get cracking!

Now remember that at the North Celestial pole you would find the Pole star (Polaris). Since it is at the Celestial North Pole, it is at a dec=90º N. Okay, that's pretty easy. If you were to stand on the Earth's North Pole, Polaris is at your zenith (or you could say that it is 90º above the horizon). See Figure 6. Being at the North Pole is not much fun, since it is so dang cold. Let's go somewhere warmer, like the Equator. If you were to stand on the Earth's Equator, Polaris is no longer at your zenith, it is on the horizon (or you could say 0º above the horizon). This is illustrated in Figure 7.

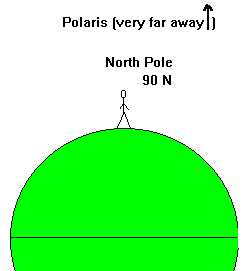
Figure 6. When you are at the North Pole, Polaris is over your head.
Figure 7. When you are the equator, Polaris is on the horizon.

Do you notice a pattern to this? What was your latitude at those locations? At the North Pole your latitude was 90º N, and Polaris was 90º above the horizon. At the Equator you latitude was 0º, and Polaris was located 0º above the horizon (actually it was right on the horizon). Wow, this is amazing! Your latitude = angle that Polaris is above the horizon. As long as you can see Polaris, you can measure its height above the horizon, and that angle will equal your latitude. Perhaps you can use this information to save your life someday when you are lost in the woods. All you would have to do would be to find Polaris, then measure its height above the horizon, and then you'd know what your latitude was. Perhaps that wouldn't really help much to save you if you were lost in the woods, but at least you'd be able to apply something you learned in this class to a real life experience. Of course in the old days, when navigators used the stars to sail the seas, the Polaris trick was really helpful. Unfortunately it only works for Polaris, since there is no star at the Celestial South Pole.

Let's try the Polaris thing for you at UNI. Remember the latitude of UNI = 42.5º N, and that means that an object at our zenith is at a declination of 42.5º N. This means that the Celestial Equator is located 42.5º south of our zenith. This is shown in Figure 8, which shows the angles and the declinations toward the northern and southern horizons. If the angle between the zenith and the Celestial Equator is 42.5º, what is the angle between the Celestial Equator and the southern horizon? 90 - 42.5 is 47.5º, so the Celestial Equator is 47.5º above the southern horizon. You may want to make note of this angle in Figure 8. Let's see what is happening on the northern side of Figure 8. The difference in declination between what's at your zenith (dec=42.5º N) and the North Celestial Pole is 90-42.5=47.5º (hey didn't we see that number somewhere else?). This is the angle between your zenith and Polaris - see where that one is in Figure 8. So what is the angle between the northern horizon and Polaris? Well, it's 90º from the zenith to the ground, and we already know one angle on the northern side, so the height of Polaris is just the difference, 90-47.5 = 42.5º.  Polaris is 42.5º above the horizon, which is what I said in the first place! If you are completely confused by this, then you better read over this section again before we go to the next one, where you will figure out some other angle problems.


Figure 8. The angles between different points on your meridian.

How to work out those annoying angle problems

Let's look at another problem, similar to the one gone over in the previous section dealing with how to use the declination values of objects. All problems like these have 3 parts -

The diagram that helps you figure out the problem, like that shown in Figure 8, shows only the northern and southern horizon. Why not the eastern or western horizon? You might want to think of these problems as meridian problems, since that is where we are looking at the objects in the sky, when they are on your meridian (I hope you didn't forget what the meridian is, because if you did, you may want to look it up again). Anyways, the diagram just shows the angles along the meridian going from the ground, the horizon, and extending upwards. You really need to get your brain around these problems, since they will definitely be on the test.

Let's go back to the set up in Figure 8, the way that the sky is oriented along your meridian as viewed from Cedar Falls. How high above the horizon would an object be if it were on the Celestial equator? That's an interesting question. First, you should remember that the Celestial equator is where the declination = 0º, and that location is shown in Figure 8. Something in that direction would be located 47.5º above the ground when it is on your meridian (also the greatest height above the horizon).

That was pretty easy; what about an object that is located at a declination of 33º N? How high above the ground is it? You have to first determine where that location is in the diagram. Here's one of the rules you'll need to remember (don't worry I'll summarize these all later): Declination is measured from the Celestial equator. So if an object has a declination of 33º N, there must be an angle equal to 33º between the object and the Celestial equator. But on which side of the Celestial Equator is the object? Is it to the left or right of the Celestial equator? Which side has the label "northern horizon"? That tells you which way is north, so you want to put the object to the north (or, in this case left) of the Celestial equator. This is shown in Figure 9. What is the height? 33º + 47.5º (the angle between the object and the Celestial equator added to the angle between the Celestial equator and the ground) = 80.5º!

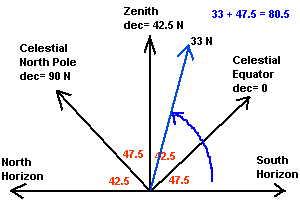Figure 9. The location of an object along your meridian which has a declination of 33º North as viewed from Cedar Falls.

Here's one that is a bit more confusing - what would the height of an object be if it has a declination of 80º N? If you follow the preceding logic, you'd find that it is 52.5º above the northern horizon. Wait a second, isn't 80º N just 10º away from the North Celestial pole? Yes, 90-10=80. Okay, so the object is 10° from the North Star, Polaris. That's fine. Now here's the tricky bit - how can you be sure of which side of Polaris the object is at? I hate to admit it, but this is a trick question, since there are actually two correct answers. How is that possible? You remember those stars that never set, circumpolar stars? That means at one time they are found above the North Celestial Pole and at another time they can be found on the other side, or below the North Celestial Pole, since they have to go in a circular path around Polaris. This is shown in Figure 10. I'll try to avoid these kinds of questions, since they are confusing.

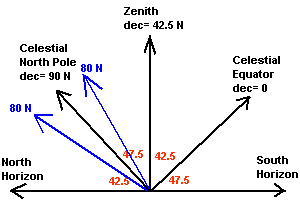Figure 10. Circumpolar objects can be found at two locations along your meridian.

Let's try another one. What would be the declination of an object that is located 30º above the Northern horizon when you are located at a latitude of 15º South. Work on this for a while and once you have an answer, or if you get completely stumped, just keep reading.

What did you get for an answer? Did you even get an answer? Let's see how you solve this - and here is where I'll summarize the way that you tackle these problems.
1. Make a drawing showing your horizon and your position.

2. Your latitude = the declination of your zenith - so you know right away one of the declination values of the sky (in this case you would put 15º S right over your head) - Figure 10a
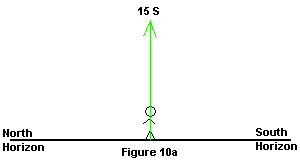3. Figure out where the Celestial equator is - is it to your north or south? You are in the southern hemisphere (you have a southern latitude), so the equator is north of you. An easy way to remember this is to see what the direction of your latitude is; the equator is in the opposite direction. The Celestial equator is to the left (north) of your zenith location - Figure 10b shows this.
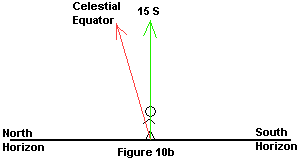4. Now put in any of the angles that you can. You know that the zenith-Celestial equator angle is 15º, since that is how it is defined (remember, declination is measured from the Celestial equator). What would be the angle between the Celestial equator line and the horizon? 90-15=75 left over - remember subtract from 90, not 100! These angles are shown in Figure 10c.
5. Now you can do anything. If you want to put in the South Celestial pole and those angles you can, but here you don't need them. Let's put the object in the picture. What was its location? In case you forgot, it is 30º above the northern horizon. Is it above the line marking the Celestial equator? No, since the Celestial equator is 75º up and 30º is much lower than that. Now you can draw it in, and you better put it below the Celestial equator. You know the angle between the object and the horizon, since that is what you're given, so put that in as well. This is shown in Figure 10d.
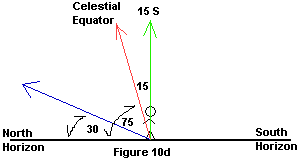6. What is its declination? How is declination measured or determined? If you haven't figured that out, I'll say it again - declination is measured from the Celestial equator. How far from the Celestial equator is the object? Hmm, the Celestial equator is 75º up, the object is 30º up, so the difference is 75-30=45º. So the declination is 45º. Is that correct? No it isn't! I forgot something. Declination is direction dependent - you have to say North or South. How do you determine if it is North or South? You need to determine if it is North or South of the Celestial equator. It's to the left of it, so that means it's North of the Celestial equator. The final answer is that the object is at a declination of 45º N. Figure 10e shows the final setup.

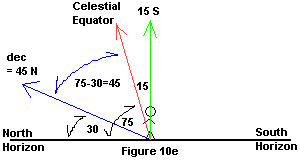If this is what you got, great! If not, well, you should work on it some more. There are more examples and practice problems available at this link.


Now that you've read this section, you should be able to answer these questions....